1. Ap Calculus Ab Unit 1 Limits Review
2. Ap Calculus Review Unit 1 Limits And Continuity
3. Unit 1 Limitsap Calculus 14th Edition
4. Unit 1 Limitsap Calculus Solver

In Class: 7.1 Limits and Convergence and 7.2a The Tangent Line Homework: Exercises 7A and 7B; page 201 7C and page 202 7D Thursday, September 6 In Class: 7.2b The Tangent Line and the Power Rule Homework: page 205:1-15 all; page 207:1, 2ab, 3bc, 5 Monday, September 10 In Class: 7.3 More Rules for Derivatives Homework: pages 209-210: 1-6, 8-12 all. Calculus Unit Plan www.pkyonge.ufl.edu Content Standards Florida Standards MAFS.912.C.1.1: Understand the concept of limit and estimate limits from graphs and tables of values. Unit 1: Functions, Graphs, and Limits This unit begins with a review of functions that should have been learned in a previous course. Specifically, we will examine the relationship between formulas and graphs of functions, as well as general properties of their graphs.

First, let’s talk about the definition of a limit.

Ap Calculus Ab Unit 1 Limits Review

Let’s say we have a function f, and we don’t know what the y-value of the function is at a certain point, say x=a.If we can follow the pattern of y-values super close to f(a), but not exactly at a, to find what the y-value should be based on the pattern, then that “prediction” is called the limit of the function f as x approaches a.

Now, we address the existence of a limit, but first we need to introduce the definition of a one-sided limit.

A left-sided limit is the y-value that a function nears, as x approaches a value from the left.

A right-sided limit is the y-value that a function nears, as x approaches a value from the right.

When we don’t mention which sided limit we are talking about, we are referring to a two-sided limit. However, a two-sided limit only exists if the left-sided limit and the right-sided limit are the same. That means a limit does not exist if the left-sided limit does not equal the right-sided limit.

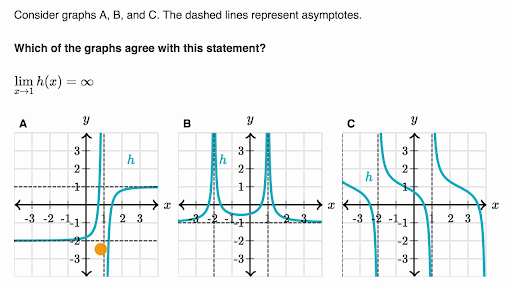
The concept of the non-existence of a limit is mostly encountered in two situations.

• First, around vertical asymptotes (one side could go to positive infinity, and the other side could go to negative infinity, or vice versa).
• Second, if we have a rational function that has a point of discontinuity (remember, this is where the denominator equals zero), and we cannot use algebra to cancel the hole and see how the function acts without the hole. Then we have to use a tabular limit or a graph to check the left and right sided limits of the function around* the hole.

It’s important to remember that even if the limit of a function at a certain point isn’t the exact value of the point (for example, if the function f acts like f(x)=x² everywhere except for at x=2, where f(2)=6, the limit of f as x approaches 2 is still 4).

We then shift gears and begin to think about limits of a rational function as x nears infinity. Suppose we have a rational function , where q(x)≠0. There are three possible cases:

Ap Calculus Review Unit 1 Limits And Continuity

If the degree of p(x) is larger than q(x), then p(x) is growing a lot faster than q(x). So, as x gets really big, p(x) is pretty much going to dwarf q(x). So the limit as x approaches infinity of f(x) is infinity.

If the degree of p(x) is smaller than the degree of q(x), then as x approaches infinity, q(x) will grow much larger much faster than p(x). Then the denominator is getting infinitely bigger than the numerator, so the limit as x approaches infinity of f(x) is zero.

Unit 1 Limitsap Calculus 14th Edition

If the degree of p(x) is equal to the degree of q(x), then the numerator and denominator are essentially growing at the same rate as x approaches infinity. In this case, the function will approach a constant. This constant is determined by the leading coefficients of p(x) and q(x).

Unit 1 Limitsap Calculus Solver

*Note: When we say “around” in reference to limits, we mean reeeeaallly close to the point, but not at the point. The tables we encounter in class and in MLP are a good example of how close we need to get. If we are looking for the limit as x approaches 2, for example, then we can use the values 1.9, 1.99, 1.999, 1.9999, and so on. But we could find values even closer to 2, like 1.999999999999999 to get a more accurate idea of what the function is doing around 2.